Optical Spin Orientation
Summary
In this project, nuclear spin fluctuations, effects of quadrupole splitting of nuclear spin states, spin-lattice relaxation and decoherence in the nuclear spin system of semiconductors and their quantum structures will be investigated. Dynamic polarization via spin-oriented electrons will be used to increase the nuclear spin polarization. Using optically-detected magnetic resonance (ODMR), optical orientation, Hanle effect, and pump-probe Faraday/Kerr rotation techniques, the nuclear spin state will be determined and its evolution in magnetic fields and under microwave and optical irradiation will be measured. The main objective of this project is the development of advanced techniques for controlling and manipulating the nuclear spin order and its back-action on the central electron spin of a localized charge carrier. The role of quadrupole splitting of nuclear spin states, which may considerably affect the nuclear-nuclear and electron-nuclear spin dynamics and carrier spin coherence, will be also studied.
Experimental setup
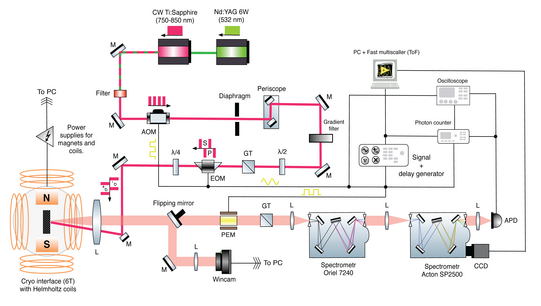
An illustration of the experiment on optical spin orientation and its detection by means of polarized luminescence is shown in the figure above. For the CW-laser excitation a pump solid-state Nd:YAG laser and a Ti:Sapphire laser are used. The Ti:Sapphire laser can be tuned in a range of 750nm – 850nm. The laser beam can be manipulated in intensity by the use of an acousto-optic modulator (AOM). The gradient filter and λ/2 help to change the intensity of light. The electro optical modulator (EOM) can rotate the polarization plane by 90° so that horizontally polarized light becomes vertically polarized. The digital pulse amplifier that can apply voltage to the crystals of the EOM with a maximum frequency of 20 MHz and the analog amplifier can apply voltage with the maximum frequency 2MHz. In combination with a λ/4 wave plate one can excite the sample with right and left handed circular polarization. The EOM and AOM can be manipulated by a four-channel function generator.
The sample under study is placed to the magneto-optical cryostat which has a reservoir for liquid helium. A vacuum pump can be connected to this reservoir in order to reach the lambda point, where helium becomes superfluid. It is possible to attain temperatures down to 1.6 K. The superconductive magnet within the cryostat is oriented in a Voight (Faraday) geometry and connected to a bipolar current source with a conversion factor of 15.1 A/T. The magnetic fields in a range of -6 … +6 Tesla can be achieved. To create an additional magnetic fields, perpendicular to the optical axis and parallel to the optical axis, two pairs of small Helmholtz coils was installed outside the cryostat. The coils which are perpendicular to the optical axis are used for compensation of parasitic magnetic fields. The coils which are parallel to the optical axis are used for applying an additional longitudinal magnetic field. For a spectral resolution of the PL a half meter spectrometer with nitrogen cooled silicon CCD is used. The avalanche photodiode shows a linear relationship between the intensity and counted photons up to a counting rate of 106 photon/secons and then the signal goes to the two channel gated photon counter.
Hanle effect
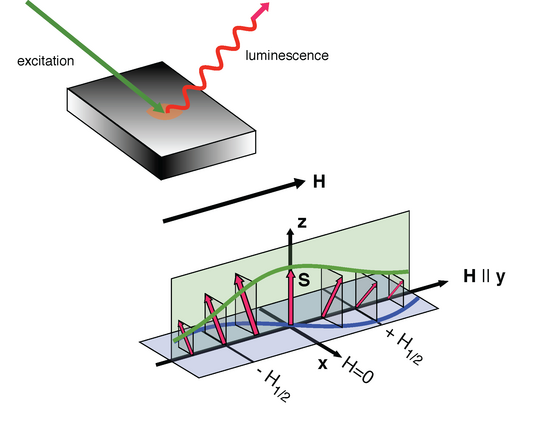
The Hanle effect is based on the depolarization of an optically excited atomic state by a magnetic field.
- The Non-Equilibrium Spin Distribution (NESD) that is initially completely aligned along z-direction.
- With the transverse magnetic field in y-direction the carriers will start precessing in the x-z plane with Lamor frequency.
- Integrating over time the transverse magnetic field will tilt the NESD in the x-direction.
- The precession will induce a dephasing in the spin ensemble, thereby reducing the overall spin- polarization and reduces the z-component of the spin polarization to half its initial value.
Description of the research and main results
A strong revival in the interest to the spin physics in semiconductors has occured in the last 20 years [1]. Because of the potentiality of using spins, instead of a carrier charge, as a new degree of freedom to develop a new spin-based electronics, “spintronics”, a large body of fundamental research was concentrated on the spin physics in semiconductor quantum dots (QDs) [2], often considered as “artificial atoms” because their energy spectrum is fully discrete. These zero-dimensional structures are useful in the context of quantum computing, because a single electron would be localized in a single QD. One of the major problem in hardware realization of quantum computation is the problem of decoherence that occurs due to interaction of the spin with an environment. In QDs, the main source of electron spin decoherence is its interaction with a large number (104–106) of nuclear spins thus representing a “nuclear spin bath” acting on the electron spin.
In many materials, the nuclei have non-vanishing spin angular momentum, and couple to the elec- trons by the isotropic Fermi-contact interaction. In the heavy atoms that typically form semiconductor QDs, the Fermi-contact interaction is greatly enhanced. As a result, polarized nuclear spins can create hyperfine fields acting on the electron spin as large as several Tesla [1].
The nuclear fields can be used to manipulate electron spins. Using resonant RF fields, it is possible to turn the nuclear field in any direction on a sphere within a few tens of microseconds. All- optical NMR has been also demonstrated in various semiconductor structures [1], but not yet perfected to the degree that would make full coherent control of nuclear fields possible. When nuclear spins are completely disordered, they still create fluctuating hyperfine fields as strong as 10–20 mT. These random fields cause a precession of electron spins, which eventually leads to electron spin relaxation [3, 4]. Because of the large number of nuclei coupled to the electron, the nuclear spin bath presents a formidable source of electron spin decoherence.
Electron spin relaxation by nuclei is accompanied by the transfer of energy and angular momentum to the nuclear spin system, causing dynamic polarization of the nuclear spins. The dynamic polarization in quantum dots is most efficient under resonant excitation of spin-forbidden optical transitions (optical solid effect) [5]. Strong hyperfine fields of polarized nuclei shift the electron spin levels, giving rise to pronounced nonlinear effects. The coupled electron-nuclear spin system can therefore exhibit multi-stability [17] and also results in stabilization of the electron spin leading to subseconds relaxation times at zero magnetic field [6]. As the hyperfine coupling with nuclear spin fluctuations is a powerful mechanism of electron spin dephasing, attempts are being made to suppress these fluctuations by generating very high levels of nuclear spin polarization, close to 100%. So far, this goal has not been achieved.
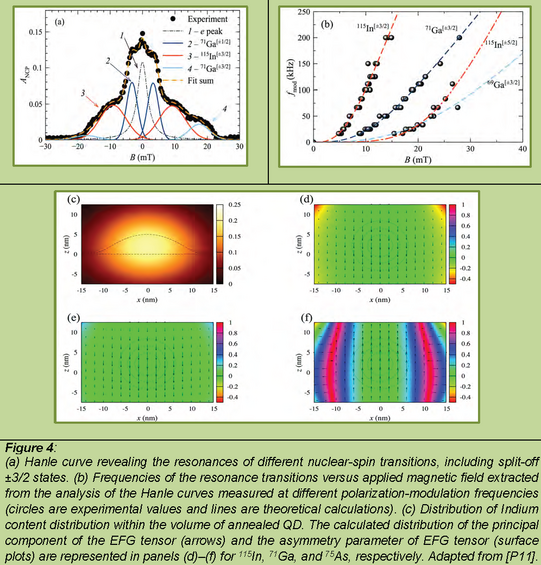
The epitaxial QDs are grown by Stranski-Krastanow mode that uses an effect of self-assembling of the crystal islands on the semiconductor substrate. Due to specifics of their formation, the built-in crystal strain relaxes to some off-zero equilibrium value. The crystal lattice remains free of lattice defects. However, the change of the positions of individual atoms from the ones in strain-free lattice results in the appearance of non-zero local electric field gradients (EFG) on the nuclear sites.
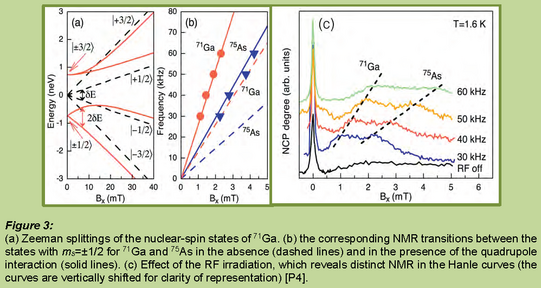
Quadrupole interaction of the nuclear spins with the EFGs drastically modifies the overall dynamics of the nuclear-spin bath. In particular, it partially eliminates the restrictions on the nuclear spin coherence of electron and nuclear spins in QDs that were pointed out in Ref. [7]. More recent studies show convincing experimental evidence for that—the nuclear spin relaxation time measured in strained QDs extends for several times versus times in strain-free dots [8]. Quadrupole effects are at the heart of many surprising effects that make spin physics in QDs different from that known in bulk and quantum wells. Such intriguing effects are, for example, the strong suppression of nuclear spin diffusion [9], the anomalous Hanle effect [10], and the nuclear-field-induced locking of excitonic transition to the incident-laser frequency [11].
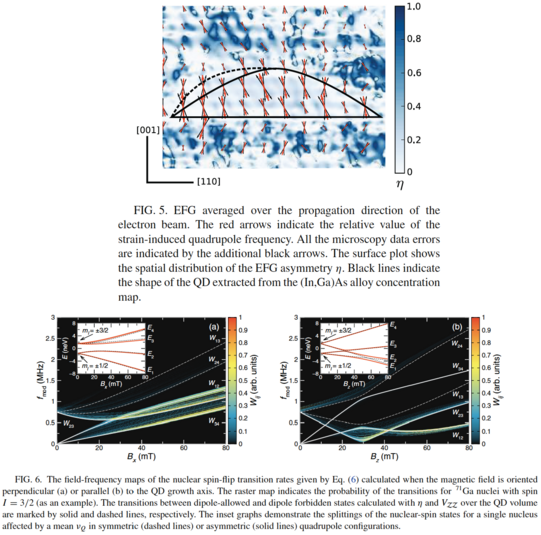
The quadrupole interaction in QDs is very inhomogeneous due to both the spatial inhomogeneity of the strain within the QD volume and due to statistical straggling of the admixture of nuclei typical for the nanostructures based on III-V solid solutions. In such conditions the Zeeman splitting of the nuclear-spin states is nonlinear thus making the spin dynamics of nuclei different in the relatively strong, i.e., larger than the local field of dipole-dipole interaction, and in weak external magnetic fields [12]. This is also supported by the modification of the optically detected NMR spectra of the QDs. A step forward to full control of the nuclear spin bath would be to develop a set of tools to overcome the inhomogeneous broadening of the NMR lines for resonant control of a much smaller subset of nuclear spins (a single nucleus in the limit case). This will enable a better understanding of the central-spin decoherence and dephasing in a QD.
References
[1] V.K. Kalevich, K.V. Kavokin, and I.A. Merkulov, Chapter on “Dynamic nuclear polarization and nuclear fields”, in Spin Physics in Semiconductors, ed. by M.I. Dyakonov (Springer, Berlin, 2008), pp. 309-346 and refs. therein.
[2] B. Urbaszek et al, Rev. Mod. Phys. 85, 79 (2013).
[3] I.A. Merkulov et al, Phys. Rev. B 65, 205309 (2002).
[4] A.V. Khaetskii et al, Phys. Rev. Lett. 88, 186802 (2002).
[5] E.A. Chekhovich et al, Phys. Rev. Lett. 104, 066804 (2010).
[6] R.V. Cherbunin et al, Phys. Rev. B 80, 035326 (2009).
[7] R.I. Dzhioev et al, Phys. Rev. Lett. 99, 037401 (2007).
[8] E.A. Chekhovich et al, arXiv:1403.1510 (unpublished).
[9] P. Maletinsky et al, Nature Physics 5, 407 (2009).
[10] O. Krebs et al, Phys. Rev. Lett. 104, 056603 (2010).
[11] A. Högele et al, Phys. Rev. Lett. 108, 197403 (2012).
[12] S.Yu. Verbin et al, Sov. Phys. JETP 114, 681 (2012).
[13] A.I. Tartakovskii et al, Phys. Rev. Lett 98, 026806 (2007).
[14] Optical orientation, Eds. F. Meier and B.P. Zakharchenja (North-Holland, Amsterdam 1984).
[15] P. S. Sokolov et al, Phys. Rev. B 93, 045301 (2016).
Current Offers For Bachelor-, Master- or PhD-Theses
Please contact us if you are interested in doing Bachelor, Master or Ph.D. on one of the topics above.
Contact
- Prof. (apl.) Dr. Dmitri Yakovlev
- Dr. Pavel Sokolov
Collaborations
- Spin Optics Laboratory, Saint-Petersburg State University
- Ioffe Physical-Technical Institute, Russian Acadamy of Sciences
- Angewandte Festkörperphysik, Ruhr-Universität Bochum



